Gravity
I've discovered that students get most excited when we show them problems that haven't been solved yet. In about twenty years, these same students might find new answers that prove our current ideas wrong. Think about it - big breakthroughs like understanding evolution, landing on the moon (Apollo missions), and creating computers all happened because people questioned old ideas.
Copyright© Schmied Enterprises LLC, 2024.
Link of the day:
Improve your workplace relationships with these 10 tips on how to become the most likable person in the office.
Here.
Link of the day:
Learn about L3Harris, a company committed to innovation and addressing the rapidly changing geopolitical environment through technologies like resilient communications and space-based missile defense.
Here.
Link of the day:
Discover the EU's new common charger rules, designed to reduce e-waste, save consumers money, and simplify charging for a wide range of devices.
Here.
Link of the day:
Explore CUDA job opportunities on Bing, connecting you with the latest openings in parallel computing and GPU programming.
Here.
Gravity is one of the most important ideas we study in physics.
I asked Claude, a smart AI helper from a company called Anthropic, to help me understand this better.
Let's work out gravity's strength using a simple math formula: g = G × M / r²
We need three numbers: • G (the gravitational constant) = 6.674 × 10⁻¹¹ N(m/kg)² • M (Earth's mass) = 5.972 × 10²⁴ kg • r (Earth's radius) = 6.371 × 10⁶ m
When we put these numbers into our formula: g = (6.674 × 10⁻¹¹) × (5.972 × 10²⁴) / (6.371 × 10⁶)² First multiply the top numbers: 6.674 × 10⁻¹¹ × 5.972 × 10²⁴ = 3.985 × 10¹⁴ Then square the bottom number: (6.371 × 10⁶)² = 4.059 × 10¹³ Finally divide: 3.985 × 10¹⁴ ÷ 4.059 × 10¹³ = 9.82 m/s²
Our answer of 9.82 m/s² is really close to the number 9.81 m/s² that we usually use. The tiny difference is because Earth isn't a perfect sphere and its density varies in different places.
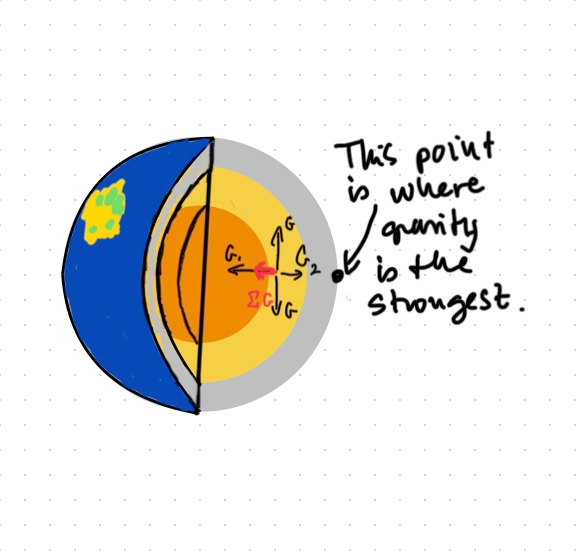
Here's something interesting: Earth's mass isn't concentrated in its center - it's spread out through the whole planet.
We created a computer model using points spread evenly through a sphere to represent Earth. We thought the average distance from any surface point to all other points would be 0.5 units, but it turned out to be 0.59 units. This means our simple gravity calculation needs some fixes.
When we add up all the tiny gravity pulls from different parts of Earth, we get a strength of 11.77 m/s² pointing toward Earth's center at sea level. Since this is different from what we actually measure (9.81 m/s²), either our gravitational constant or Earth's mass estimate needs adjusting.
To be more accurate, we need to consider that different materials inside Earth have different densities. Scientists thought Earth had an iron core and fluid mantle, but our calculations show something surprising - there's zero gravity at Earth's center!
The gravity force gradually gets stronger as you move from the center to the surface, where it reaches 9.81 m/s². Since heavy liquids flow toward stronger gravity, we might expect heavy metals like iron build up closer to the surface, not in the core as previously thought.
But there's another possibility: if Earth's core is solid iron, and different layers move against each other, this could explain Earth's magnetic field. The movement of hot liquid rock in the mantle, pushed by Earth's rotation (Coriolis force), could keep the inside of Earth moving explaining earthquakes and volcanic activity.
Reference